Rule of 9s chart. Mastering the Rule of Nines: A Comprehensive Guide to Calculating Burn Percentage in Adults
What is the Rule of Nines? How can it be used to calculate the total body surface area percentage that is burned in adult patients? Find the answers to these questions and more in this comprehensive guide.
Understanding the Rule of Nines in Burn Percentage Calculation
The Rule of Nines is a widely used method for estimating the total body surface area (TBSA) that has been burned in adult patients. This rule divides the body into sections, each representing approximately 9% of the total body surface area. By identifying the specific areas of the body affected by burns and applying the corresponding percentages, healthcare professionals can accurately calculate the overall percentage of the TBSA that has been burned.
Applying the Rule of Nines: NCLEX Quiz Questions and Answers
To help healthcare professionals, particularly nursing students, solidify their understanding of the Rule of Nines, we’ve compiled a series of NCLEX-style quiz questions. These questions cover various scenarios involving burn injuries in adults, and the answers demonstrate how to apply the Rule of Nines to calculate the total body surface area percentage that is burned.

Scenario 1: 25-Year-Old Female Patient
A 25-year-old female patient has sustained burns to the back of the right arm, posterior trunk, front of the left leg, anterior head and neck, and perineum. Using the Rule of Nines, calculate the total body surface area percentage that is burned.
Answer: The correct answer is B. 37%. The back of the right arm (4.5%), posterior trunk (18%), front of the left leg (9%), anterior head and neck (4.5%), and perineum (1%) add up to a total of 37% of the TBSA that is burned.
Scenario 2: 68-Year-Old Male Patient
A 68-year-old male patient has partial-thickness burns to the front and back of the right and left leg, front of the right arm, and anterior trunk. Using the Rule of Nines, calculate the total body surface area percentage that is burned.
Answer: The correct answer is C. 58.5%. The front and back of the right and left leg (36%), front of the right arm (4.5%), and anterior trunk (18%) add up to a total of 58.5% of the TBSA that is burned.
Scenario 3: 35-Year-Old Male Patient
A 35-year-old male patient has full-thickness burns to the anterior and posterior head and neck, front of the left leg, and perineum. Using the Rule of Nines, calculate the total body surface area percentage that is burned.

Answer: The correct answer is D. 19%. The anterior and posterior head and neck (9%), front of the left leg (9%), and perineum (1%) add up to a total of 19% of the TBSA that is burned.
Scenario 4: 66-Year-Old Female Patient
A 66-year-old female patient has deep partial-thickness burns to both of the legs on the back, front and back of the trunk, both arms on the front and back, and front and back of the head and neck. Using the Rule of Nines, calculate the total body surface area percentage that is burned.
Answer: The correct answer is C. 81%. Both of the legs on the back (18%), front and back of the trunk (36%), both arms on the front and back (18%), and front and back of the head and neck (9%) add up to a total of 81% of the TBSA that is burned.
Scenario 5: 58-Year-Old Female Patient
A 58-year-old female patient has superficial partial-thickness burns to the anterior head and neck, front and back of the left arm, front of the right arm, posterior trunk, front and back of the right leg, and back of the left leg. Using the Rule of Nines, calculate the total body surface area percentage that is burned.

Answer: The correct answer is A. 63%. The anterior head and neck (4.5%), front and back of the left arm (9%), front of the right arm (4.5%), posterior trunk (18%), front and back of the right leg (18%), and back of the left leg (9%) add up to a total of 63% of the TBSA that is burned.
Scenario 6: 35-Year-Old Male Patient
A 35-year-old male patient has superficial partial-thickness burns to the anterior right arm, posterior left leg, and anterior head and neck. Using the Rule of Nines, calculate the total body surface area percentage that is burned.
Answer: The correct answer is C. 18%. The anterior right arm (4.5%), posterior left leg (9%), and anterior head and neck (4.5%) add up to a total of 18% of the TBSA that is burned.
Understanding and correctly applying the Rule of Nines is a crucial skill for healthcare professionals, particularly nurses, when caring for patients with burn injuries. By mastering this technique, you can accurately assess the extent of the burn and determine the appropriate treatment plan, including fluid resuscitation using the Parkland Burn Formula. Remember to review the lecture on the Rule of Nines before attempting the quiz to ensure you have a solid foundation in this important concept.

Rule of Nines NCLEX Quiz Questions
Rule of Nines NCLEX questions quiz for the adult patient who has experienced burns. The Rule of Nines is used to calculate the total body surface for a patient who has experienced burns.
Based on the percentage of total body surface area that is burned, the provider can calculate the amount of fluid replacement (Lactated Ringer’s) the patient will need during the first 24 hours after the burn using the Parkland Burn Formula. As the nurse it is essential you are familiar with the Rule of Nines calculation.
Don’t forget to watch the lecture on Rule of Nines before taking the quiz.
Rule of Nines NCLEX Quiz Questions
This quiz will test your knowledge on using the Rule of Nines for burns in the adult.
- 1. A 25 year old female patient has sustained burns to the back of the right arm, posterior trunk, front of the left leg, anterior head and neck, and perineum.
 Using the Rule of Nines, calculate the total body surface area percentage that is burned?*
Using the Rule of Nines, calculate the total body surface area percentage that is burned?*- A. 46%
- B. 37%
- C. 36%
- D. 28%
- 2. A 68 year old male patient has partial thickness burns to the front and back of the right and left leg, front of right arm, and anterior trunk. Using the Rule of Nines, calculate the total body surface area percentage that is burned?*
- A. 40.5%
- B. 49.5%
- C. 58.5%
- D. 67.5%
- 3. A 35 year old male patient has full thickness burns to the anterior and posterior head and neck, front of left leg, and perineum. Using the Rule of Nines, calculate the total body surface area percentage that is burned?*
- A. 37%
- B. 14.5%
- C.
 29%
29% - D. 19%
- 4. A 66 year old female patient has deep partial-thickness burns to both of the legs on the back, front and back of the trunk, both arms on the front and back, and front and back of the head and neck. Using the Rule of Nines, calculate the total body surface area percentage that is burned?*
- A. 72%
- B. 63%
- C. 81%
- D. 45%
- 5. A 58 year old female patient has superficial partial-thickness burns to the anterior head and neck, front and back of the left arm, front of the right arm, posterior trunk, front and back of the right leg, and back of the left leg. Using the Rule of Nines, calculate the total body surface area percentage that is burned?*
- A. 63%
- B. 81%
- C. 72%
- D.
 54%
54%
- 6. A 35 year old male patient has superficial partial-thickness burns to the anterior right arm, posterior left leg, and anterior head and neck. Using the Rule of Nines, calculate the total body surface area percentage that is burned?*
- A. 36%
- B. 9%
- C. 18%
- D. 29%
1. A 25 year old female patient has sustained burns to the back of the right arm, posterior trunk, front of the left leg, anterior head and neck, and perineum. Using the Rule of Nines, calculate the total body surface area percentage that is burned?
A. 46%
B. 37%
C. 36%
D. 28%
The answer is B. Back of right arm (4.5%), posterior trunk (18%), front of left leg (9%), anterior head and neck (4.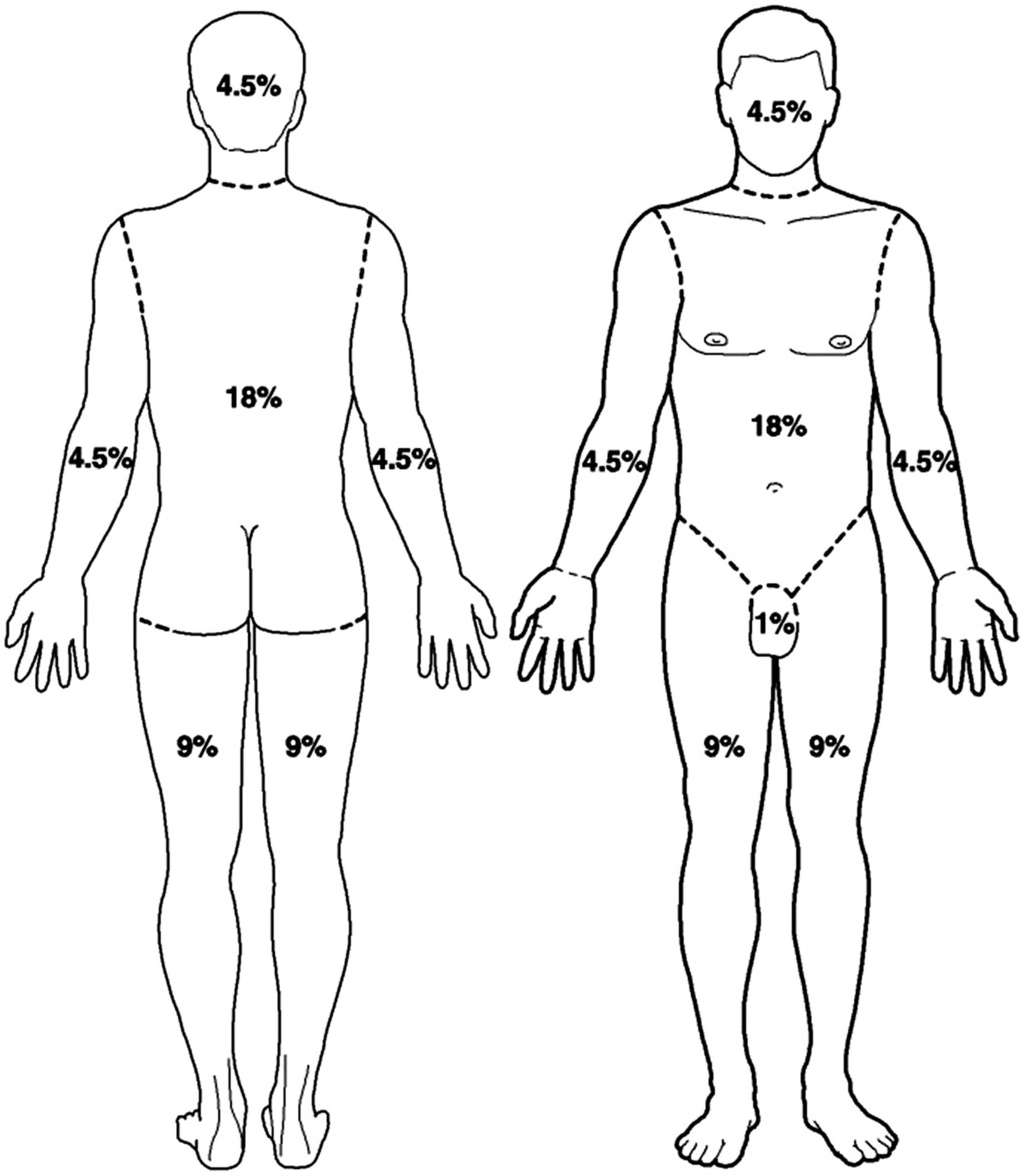 5%) and perineum (1%) which equals 37%.
5%) and perineum (1%) which equals 37%.
2. A 68 year old male patient has partial thickness burns to the front and back of the right and left leg, front of right arm, and anterior trunk. Using the Rule of Nines, calculate the total body surface area percentage that is burned?
A. 40.5%
B. 49.5%
C. 58.5%
D. 67.5%
The answer is C. Front and back of right and left leg (36%), front of right arm (4.5%), anterior trunk (18%) which equals 58.5%.
3. A 35 year old male patient has full thickness burns to the anterior and posterior head and neck, front of left leg, and perineum. Using the Rule of Nines, calculate the total body surface area percentage that is burned?
A. 37%
B. 14.5%
C. 29%
D. 19%
The answer is D. Anterior and posterior head and neck (9%), front of left leg (9%), perineum (1%) which equals 19%.
4. A 66 year old female patient has deep partial-thickness burns to both of the legs on the back, front and back of the trunk, both arms on the front and back, and front and back of the head and neck.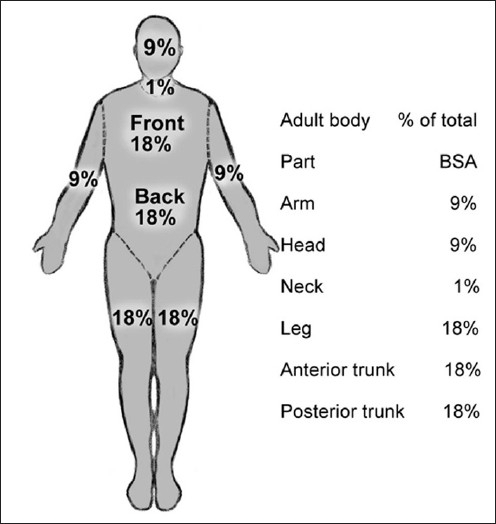 Using the Rule of Nines, calculate the total body surface area percentage that is burned?
Using the Rule of Nines, calculate the total body surface area percentage that is burned?
A. 72%
B. 63%
C. 81%
D. 45%
The answer is C. Both of the legs on the back (18%), front and back of the trunk (36%), both arms on the front and back (18%), front and back of the head and neck (9%) which equals 81%.
5. A 58 year old female patient has superficial partial-thickness burns to the anterior head and neck, front and back of the left arm, front of the right arm, posterior trunk, front and back of the right leg, and back of the left leg. Using the Rule of Nines, calculate the total body surface area percentage that is burned?
A. 63%
B. 81%
C. 72%
D. 54%
The answer is A. Anterior head and neck (4.5%), front and back of the left arm (9%), front of the right arm (4.5%), posterior trunk (18%), front and back of the right leg (18%), back of the left leg (9%) which equals 63%.
6. A 35 year old male patient has superficial partial-thickness burns to the anterior right arm, posterior left leg, and anterior head and neck.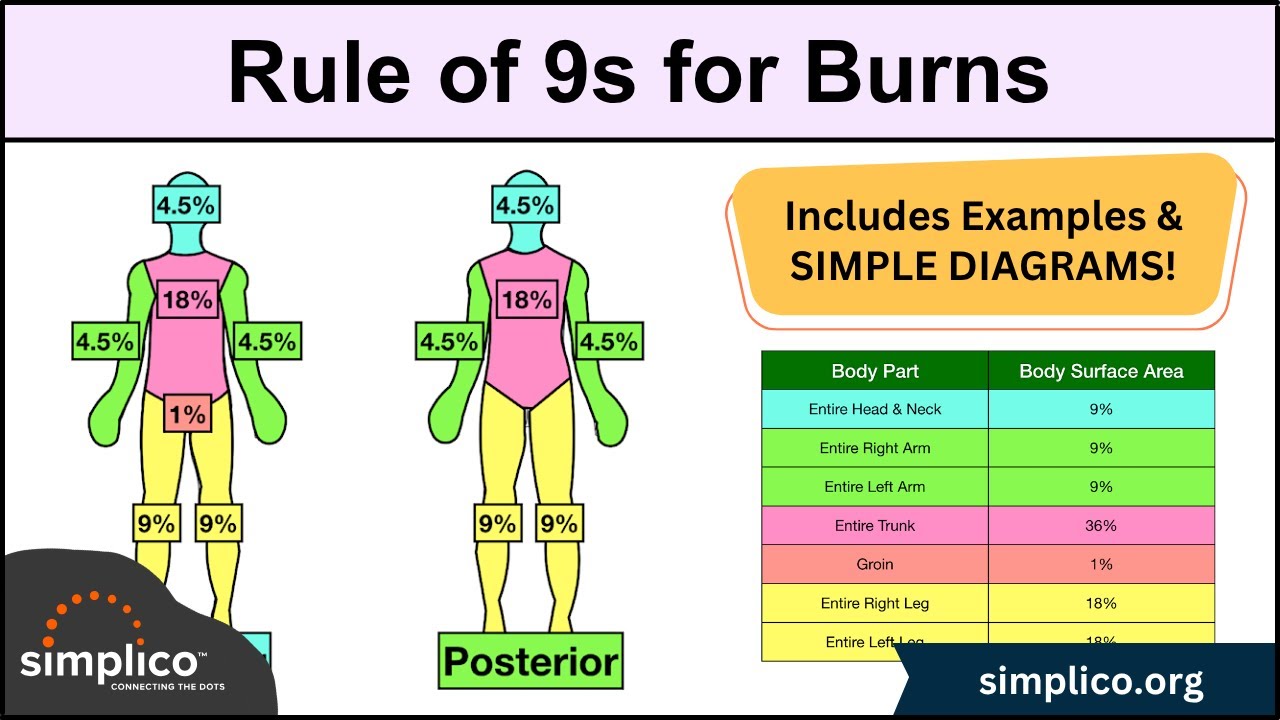 Using the Rule of Nines, calculate the total body surface area percentage that is burned?
Using the Rule of Nines, calculate the total body surface area percentage that is burned?
A. 36%
B. 9%
C. 18%
D. 29%
The answer is C. Anterior right arm (4.5%), posterior left leg (9%), and anterior head and neck (4.5%) which equals 18%.
More NCLEX Quizzes
Don’t forget to tell your friends about this quiz by sharing it your Facebook, Twitter, and other social media. You can also take more fun nursing quizzes.
*Disclaimer: While we do our best to provide students with accurate and in-depth study quizzes, this quiz/test is for educational and entertainment purposes only. Please refer to the latest NCLEX review books for the latest updates in nursing. This quiz is copyright RegisteredNurseRn.com. Please do not copy this quiz directly; however, please feel free to share a link to this page with students, friends, and others.
Burn Percentage With Charts & Images
Posted by
—
in Diseases
—
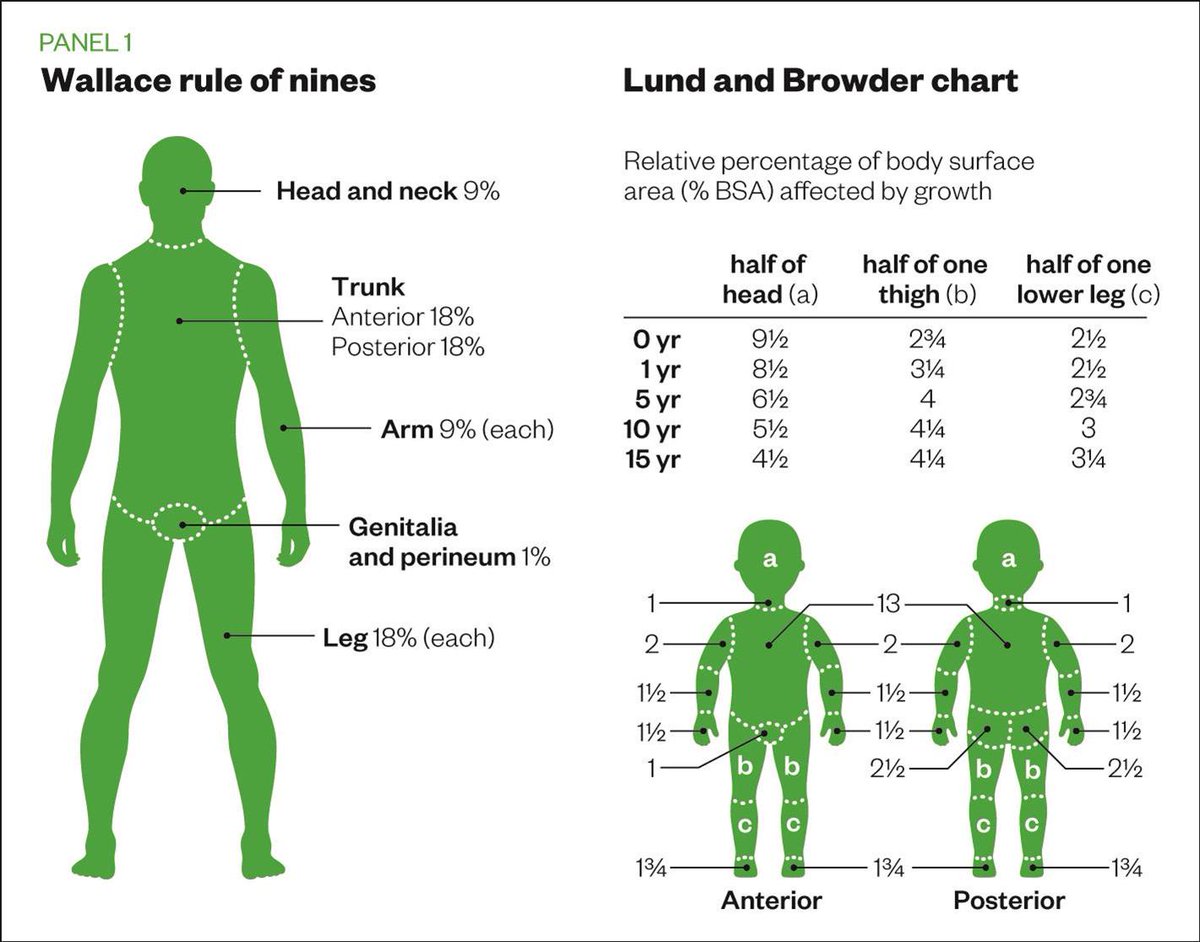
The Rule of Nines determines the percentage of burns which is useful to direct treatment solution choices such as fluid resuscitation and will become a part of the ideas to figure out transport to a burning part.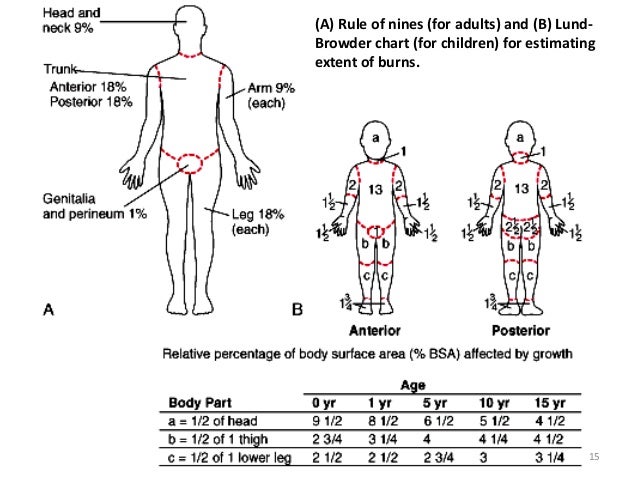 Rules of nines is a strategy of estimating the degree of burn, expressed as a percentage of overall body surface area.
Rules of nines is a strategy of estimating the degree of burn, expressed as a percentage of overall body surface area.
Using this approach, the whole body is separated into segments of 9 per cent, as well as multiples of 9 per cent, each: head and neck, 9 per cent; anterior trunk area, 18 per cent; posterior trunk area, 18 per cent; upper limbs, 18 per cent; lower limbs, 36 per cent; genitalia and perineum, 1 per cent. The rules of nines are adequate about adults but limits for differences in percentage in children, for that the Lund and Browder classification is generally used.
Topics Covered
What is the Rule of Nines (Definition)
Rules of nines: A strategy practiced in calculating entire body area involved with burns, wherein values of 9% 18% of area are allotted to areas in the adult the following: Head and neck, 9%; anterior thorax, 18%; posterior thorax, 18%; arms, 9% each; legs, 18% each; and perineum, 1%.
Rules of Nine Burns in Child
The ‘Rule of 9’s’ approach is very imprecise for estimating the burnt area in young children simply because the infant or young child’s head, as well as lower extremities, signify different percentages of the area as compared to a fully grown.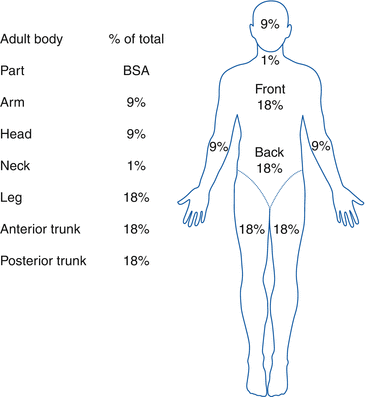 Burns above 15% in an adult, over 10% in the child, or even any specific burn taking place in the still very young or older are dangerous.
Burns above 15% in an adult, over 10% in the child, or even any specific burn taking place in the still very young or older are dangerous.
Wallace Rules of Nine
The Wallace Rule of Nines is useful in approximating the percentage of a patient that is burned. It smears a percent of Body Surface Area, repeatedly a multiple of 9, for various parts of the body as follows:
- 9% Entire head
- 9% Complete left arm
- 9% Complete right arm
- 9% Entire chest
- 9% Entire abdomen
- 18% Complete back
- 1% Groin
- 18% Complete left leg
- 18% Complete right leg
Rules of Nine in Adults
The rules of nines determine the % of burns that are familiar with benefit direct remedy choices including fluid resuscitation and turns out to be a part of the ideas to figure out transmit to a burn area.
You might approximate the entire body area on a matured adult that has already been burned by applying multiples of 9.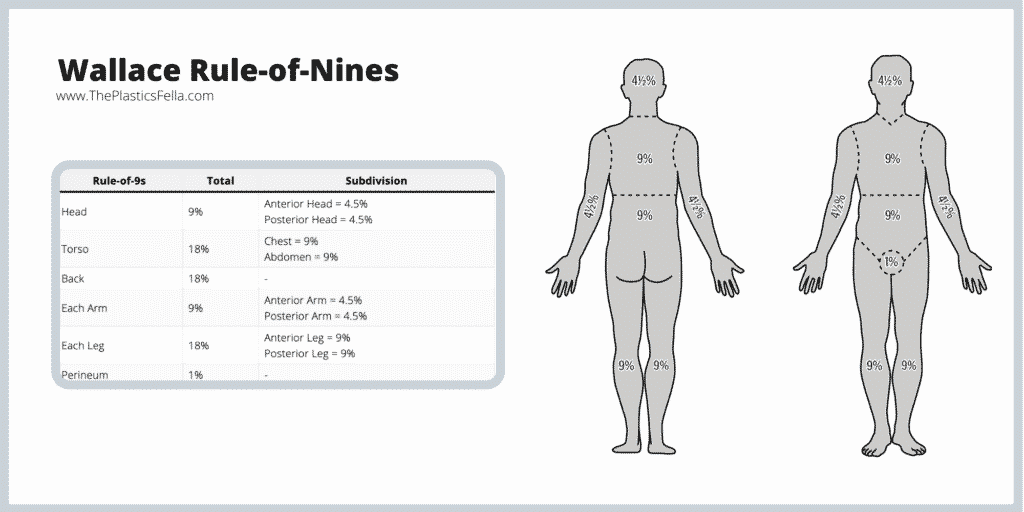 An adult which has been burned, the % of the entire body related could be computed as below:
An adult which has been burned, the % of the entire body related could be computed as below:
For example, when both the hands (18% x 2 = 36%), the groin (1%) along with the front chest (9%) and abdominal area (9%) were burned, this could involve 55% of the entire body.
Other Posts:
- Elephantitis Symptoms, Causes, Treatment, Prevention
- Kwashiorkor Symptoms and Causes
- Bruised Ribs Symptoms and Causes
- Pancoast Tumor: Symptoms, Causes, Diagnosis, Treatment
- Hernia: Symptoms, Causes, Prevention, and Treatment
You May Also Like
Building and solving graphs of Functions
We will teach you how to build any graphs of functions
Start learning
515.1K
At school in mathematics, we often work with numbers and formulas than with drawings. It’s time to fix it! To prepare for the Unified State Examination, function graphs will definitely come in handy for us – we’ll talk about this.
Function concept
Function is the dependency of y on x , where x is the independent variable or function argument and y – dependent variable or function value. A function is a correspondence between two sets, with each element of the first set corresponding to one and only one element of the second set.
To specify a function means to define a rule according to which the corresponding values of the function can be found from the values of the independent variable. Here are the ways you can set it:
Tabular method – helps you quickly determine specific values without additional measurements or calculations.
The graphic method is visual.
Analytical method – through formulas.
 Compact, and you can calculate the function for an arbitrary value of the argument from the domain.
Compact, and you can calculate the function for an arbitrary value of the argument from the domain.Verbal method.
Function scope is the set of all valid values of the argument (variable x). Geometrically, this is the projection of the function graph onto the Ox axis.
For example, for a view function, the scope looks like this
x ≠ 0 because you can’t divide by zero. You can write it like this: D (y): (-∞; 0) ⋃ (0; +∞).
The range of the function is the set of all values that the function takes on the domain of definition. Geometrically, this is the projection of the function graph onto the Oy axis.
For example, the natural range of the function y = x 2 is all numbers greater than or equal to zero. You can write like this: E (y): [0; +∞).
You can write like this: E (y): [0; +∞).
Find out what professions of the future will suit you
Take the test and we will show you who you can become, and we will also send you a detailed guide on how to realize yourself right now
The concept of a graph of a function )
, whose coordinates are related by the relation y = f(x). The very equality y = f(x) is called the equation of this graph .
Function graph is a set of points (x; y), where x is argument and y is is the function value that matches the given argument.
Simply put, the graph of a function shows the set of all points whose coordinates can be found by simply substituting any numbers into the function instead of x .
For example, let’s take the simplest function, in which the argument is equal to the value of the function, that is, y = x .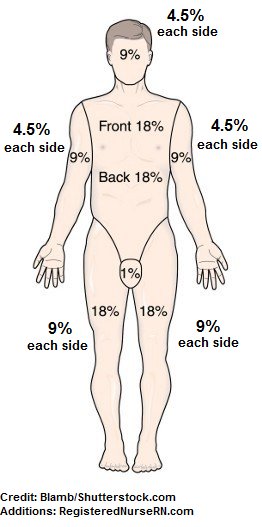
In this case, we do not have to calculate the value of the function for each argument, since they are equal, therefore, for all points of our graph, the abscissa will be equal to the ordinate.
Mark any three points on the coordinate plane, for example: L (-2; -2), M (0; 0) and N (1; 1).
If we sequentially connect the marked points from the smallest value of the argument to the largest, then we get a straight line. So the graph of the function y = x is a straight line. On the graph, it looks like this:
The inscription on the drawing y = x is the equation of the graph. It is convenient to put an inscription with an equation on the drawing so as not to get confused when solving problems.
It is important to note that a straight line is infinite in both directions. Although we call a part a straight graph of a function, in fact, only a small part of the graph is shown in the drawing.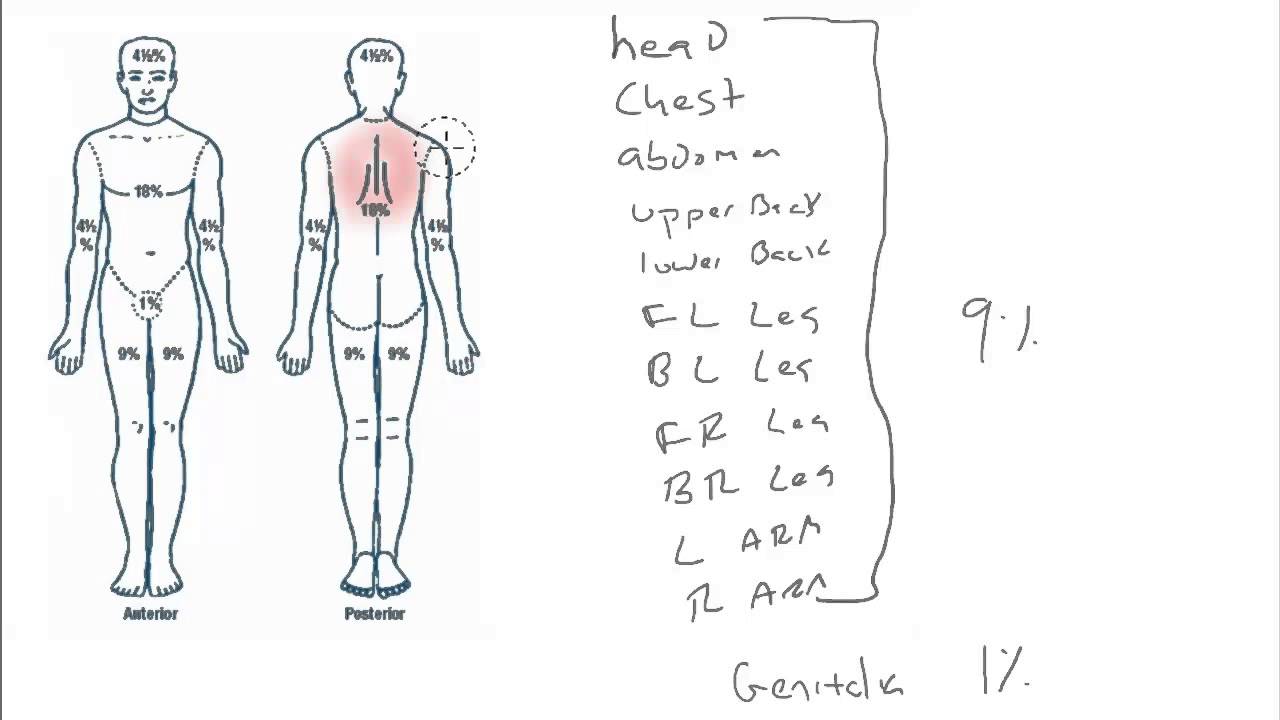
Remember!
It is not necessary to make a drawing on a whole notebook sheet, you can choose a scale that is convenient for you, which will reflect the essence of the task.
Investigation of the function
Important points of the graph of the function y = f(x):
stationary and critical points;
extremum points;
function zeros;
function break points.
Stationary points are points where the derivative of the function f(x) is equal to zero.
Critical points are points where the derivative of the function f(x) is equal to zero or does not exist. Stationary points are a subset of the set of critical points.
Extremum in mathematics is the maximum or minimum value of a function on a given set.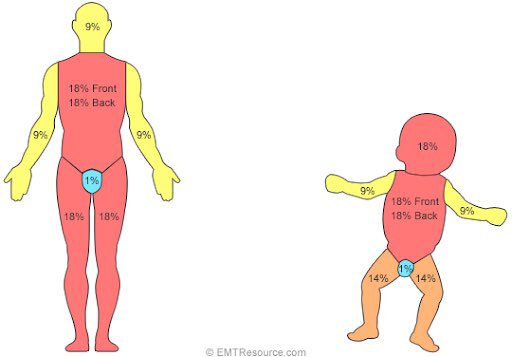 The point at which the extremum is reached is called extremum point . Accordingly, if the minimum is reached, the extremum point is called the minimum point, and if the maximum is reached, the maximum point.
The point at which the extremum is reached is called extremum point . Accordingly, if the minimum is reached, the extremum point is called the minimum point, and if the maximum is reached, the maximum point.
Function zeros are the values of the argument at which the function value is equal to zero.
The asymptote is a straight line that has the property that the distance from a point of the graph of a function to this straight line tends to zero with an unlimited distance from the origin of the graph point. According to the methods of finding them, three types of asymptotes are distinguished: vertical, horizontal, oblique.
The function is continuous at point k, if the limit of the function at the given point is equal to the value of the function at this point:
If the function f(x) is not continuous at the point x = a, then f(x) is said to have break at this point .
If we need to build a graph of an unfamiliar function, when it is impossible to imagine the form of the graph in advance, it is useful to apply the scheme of studying the properties of the function. It will help you get an idea of the graph and start plotting by points.
Function plotting scheme:
Find the domain of the function.
Find the range of allowed values of the function.
Check if the function is even or odd.
Check if the function is periodic.
Find the point of intersection with the OY axis (if any).
Calculate the derivative and find the critical points, determine the intervals of increase and decrease.

Intervals of sign constancy.
Asymptotes.
On the basis of the study, build a graph of the function.
Plotting a function
To understand how to plot a function, practice with examples or use the online simulator.
Task 1. Graph the function
How to solve:
Simplify the formula of the function:
for x ≠ -1.
The graph of the function is a straight line y = x – 1 with a punched out point M (-1; -2).
Task 2. Construct a graph of the function
How to solve it: 3 to the right at x and 2 up at y and stretched by 10 times compared to the graph of the function
Task 3.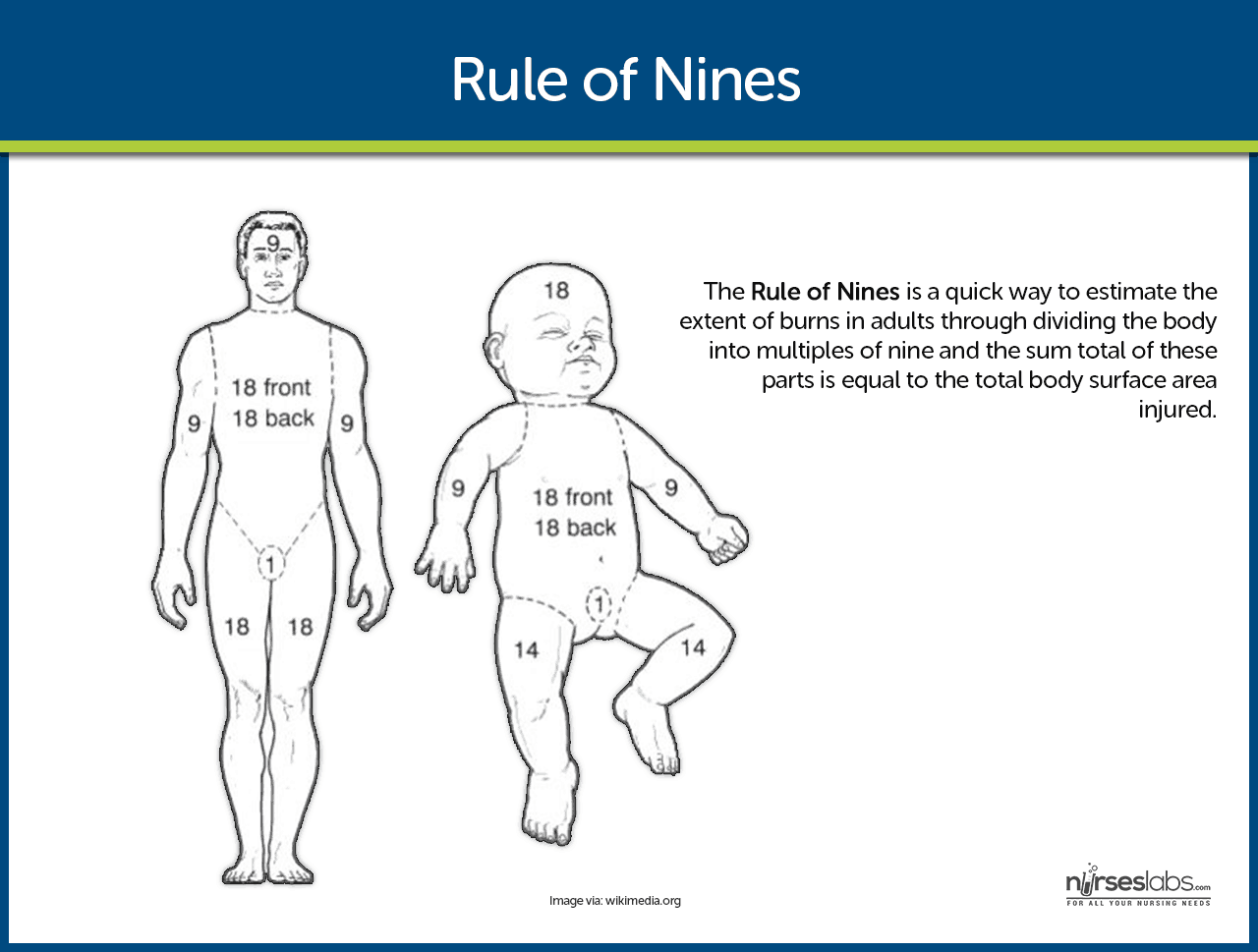 Construct function graphs:
Construct function graphs:
0003
b) y = -x + 2
c) y = 2x
d) y = -1
How we solve:
Let’s use the method of constructing linear functions “by points”.
a) y = 3x – 1
| x | and |
| 0 | -1 |
| 1 | 2 |
As you can see, k = 3 > 0 and the angle of inclination to the Ox axis is acute, b = -1 is the offset along the Oy axis.
b) y = -x + 2
| x | and |
| 0 | 2 |
| 1 | 1 |
k = -1 > 0 and b = 2, we can draw similar conclusions as in the first paragraph.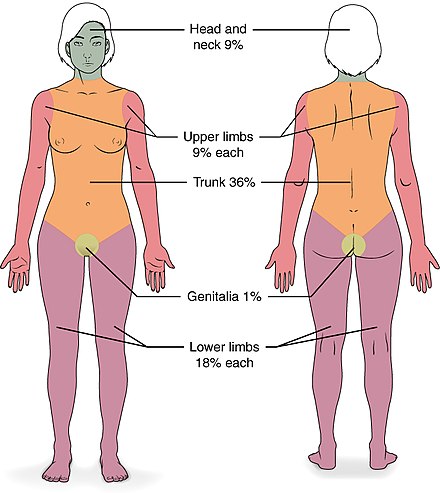
c) y = 2x
| x | and |
| 0 | 0 |
| 0 | 2 |
k = 2 > 0 — the angle of inclination to the Ox axis is acute, b = 0 — the graph passes through the origin.
d) y = -1
k = 0 is a constant function, the straight line passes through the point b = -1 and is parallel to the Ox axis.
Task 4. Based on the type of graph, determine the signs of the coefficients of the general form of the function y = ax 2 + bx + c.
How to solve:
Recall how the parameters a, b and c determine the position of the parabola.
Branches down, hence a < 0.
Point of intersection with the Oy axis – c = 0.
Vertex coordinate
Branches up, hence a > 0.
Point of intersection with the Oy axis – c > 0.
Vertex coordinate, as an unknown number when divided by a positive gives a negative result, then this number is negative, therefore, b > 0.
Branches down, hence a < 0.
Point of intersection with the Oy axis – c > 0.
Vertex coordinate, as an unknown number when divided by a negative gives a positive result, then this number is negative, therefore, b < 0.
Task 5. Construct function graphs:
2 e)
How do we decide :
Graphs can be built using elementary transformations.
If the graph of the function y = f(x) is constructed, then for a > 0 the following graphs are obtained by shifting the graph f(x).
y = f(x) + a — the graph of the function y = f(x) shifts a units up;
y = f(x) − a — the graph of the function y = f(x) is shifted down by a units;
y = f(x + a) — the graph of the function y = f(x) is shifted by a units to the left;
y = f(x − a) — the graph of the function y = f(x) shifts a units to the right.
Graph transformation by type y = mf(x): y = f(x) → y = mf(x), where m is a positive number.
If m > 1, then such a graph transformation is called stretching along the y-axis with a factor m.
If m < 1, then such a transformation of the graph is called compression to the x-axis with a factor of 1/m.

a)
Transformation into a single action like f(x) + a.
y = x²
Shift the graph up by 1:
y = x² + 1
b)
Transformation into one action like f(x – a).
Shift the graph to the right by 1:
c) y = (x – 1)² + 2
In this example, there are two transformations, we will perform them in the order of actions: first, the actions in brackets f (x – a), then addition f(x) + a.
y = x²
Shift the chart to the right by 1:
y = (x – 1)²
Shift the chart up by 2:
y = (x – 1)² + 2
d)
9 0012 One step conversion type
y = cos(x)
We stretch the graph 2 times from the y-axis along the x-axis:
e)
-f(x).

 29%
29%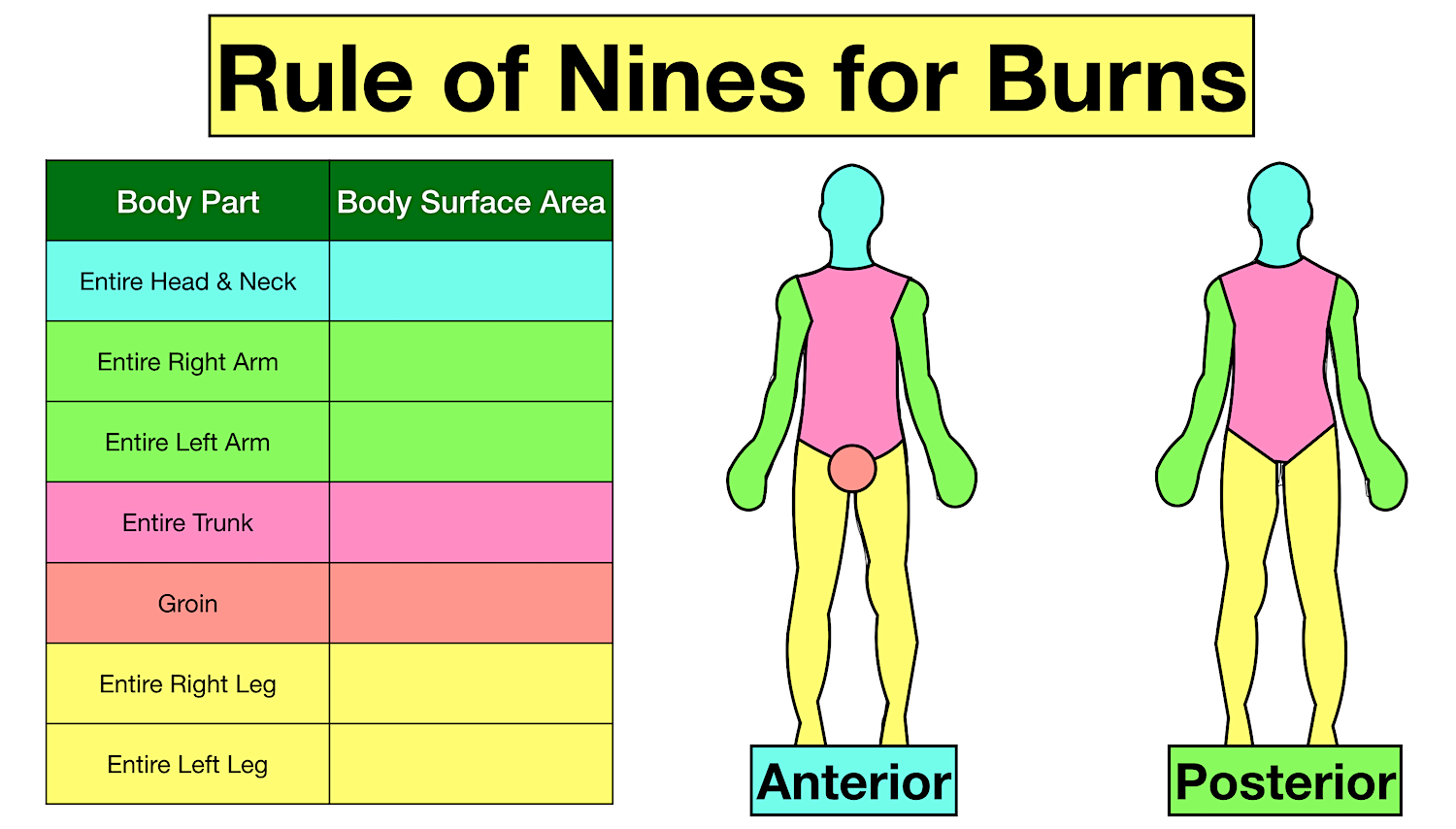 54%
54% Compact, and you can calculate the function for an arbitrary value of the argument from the domain.
Compact, and you can calculate the function for an arbitrary value of the argument from the domain.
